In a friend's get together party on New Year's Eve, a power cut takes place unexpectedly. When the lights go live again, it is found that Joseph, one of the friends has been stabbed to death. Since no one else had the access to the party, the killer is one of the friends only.
Police are called and they search the surroundings for any evidence, but can't find any. Upon checking the pockets of Joseph's pants, they find a pocket calendar and they find out that it has been marked and can also find some numbers written on it.
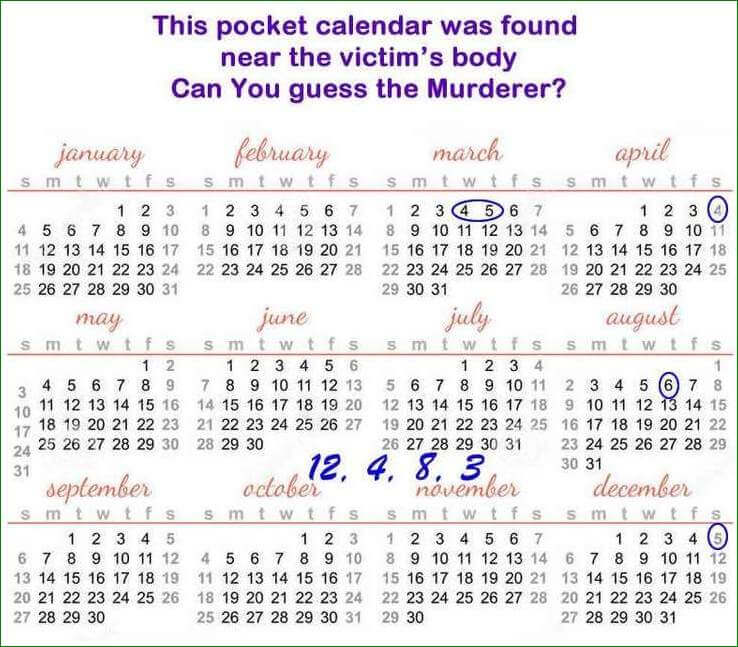
You can see the calendar attached with this question.
You don't know the name of the friends who were there at the party. Can you still decipher the name of the killer through the picture?
Solution:
The numbers that have been written over the calendar are:
12, 4, 8 and 3
A few numbers have been circled under particular months. The curious thing is that the numbers that have been written are the same number of corresponding months under which the dates have been marked.
What we can judge by the overall calendar is that the dates that have been marked may refer to the letters at that position in the month's name. In addition, the written numbers can be the position of those letters. Let's simplify below:
12th month is December
The number marked under December = 5
The fifth letter of December = M
4th month is April
The number marked under April = 4
The fourth letter of April = I
8th month is August
The number marked under August is 6
The sixth letter of August = T
3rd month is March
The numbers marked under March are 4 and 5
The fourth and fifth letter of March = C and H
When you combine the letters, you get the name as MITCH.
Therefore, Mitch is the killer.








 Submit your Email Address to get latest post directly to your inbox.
Submit your Email Address to get latest post directly to your inbox.